ESPE2016 Free Communications Growth: Clinical (6 abstracts)
Birth Characteristics Explain One Third of Expected Deaths in rhGH-treated Patients Diagnosed with IGHD, ISS & SGA
Kerstin Albertsson-Wikland a , Anton Mårtensson a, , Lars Sävendahl c , Aimon Niklasson d , Peter Bang e , Jovanna Dahlgren d , Jan Gustafsson f , Berit Kriström g , Svante Norgren c , Nils-Gunnar Pehrsson b & Anders Odén b,
aDepartment of Physiology/Endocrinology, Institute of Neuroscience and Physiology, The Sahlgrenska Academy at University of Gothenburg, Gothenburg, Sweden; bStatistiska Konsultgruppen, Gothenburg, Sweden; cDepartment of Women’s and Children’s Health, Karolinska Institutet, and Pediatric Endocrinology Unit, Karolinska University Hospital, Stockholm, Sweden; dGothenburg Pediatric Growth Research Center (GP-GRC), Department of Pediatrics, Institute of Clinical Sciences, The Sahlgrenska Academy at University of Gothenburg, Gothenburg, Sweden; eDepartment of Clinical and Experimental Medicine, Division of Pediatrics, University of Linköping, Linköping, Sweden; fDepartment of Women’s and Children’s Health, Uppsala University, Uppsala, Sweden; gDepartment of Clinical Science, Pediatrics, Umeå University, Umeå, Sweden; hDepartment of Mathematical Sciences, Chalmers University of Technology, Gothenburg, Sweden
Background: That mortality is not increased in rhGH-treated patients when adjusting for birth characteristics was recently published (1). When applying a developed mortality model of the general population, the observed and expected deaths in rhGH-treated IGHD, ISS and SGA patients (n=3847) where 21 and 21.99, respectively. The model includes gender, age, calendar year, gestational age (GA), birth lengthSDS (BLSDS), birth weightSDS (BWSDS) and congenital malformations. When the model only adjusted for gender, age and calendar year (as for traditional SMR) the number of expected deaths was 14.68.
Objective and hypotheses: How much of the expected deaths in rhGH-treated patients can be explained by the different birth characteristics.
Method: Multiple stepwise regressions of the Poisson mortality model was performed, evaluating the results of different combinations of GA, BLSDS and BWSDS in addition to gender, age and calendar year with interactions between variables included as in the published model (1). Malformations were not included in these models causing the exact numbers to differ slightly (<2%) from previous calculations (1).
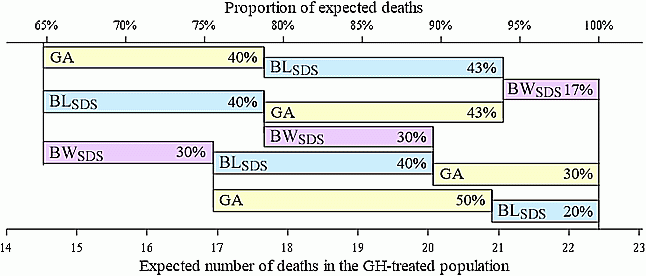
Results: As much as 35% of the expected number of deaths (7.9/22.4) can be explained by different combinations of GA, BLSDS and BWSDS as visualized in the figure below. Depending on the order the variables are included, the number of deaths explained by GA became 30,40,43,50%; explained by BLSDS became 20,40,43%; and explained by BWSDS became 17,30% (see figure).
Conclusion: As much as 35% of expected deaths among GH-treated children can be explained by various combinations of birth characteristics; GA, BLSDS and BWSDS; valuable information when validating the Swedish mortality model in countries missing information. References: 1) Albertsson-Wikland, K et al. JCEM 2016:101. doi: 10.1210/jc.2015-3951
Article tools
My recent searches
My recently viewed abstracts
Authors
ESPE Abstracts
© Bioscientifica 2024 |
Privacy policy |
Cookie settings
BiosciAbstracts
Bioscientifica Abstracts is the gateway to a series of products that provide a permanent, citable record of abstracts for biomedical and life science conferences.
Find out more